This introduction will be great math homework help for fractions. You’ll get a quick refresher on fraction fundamentals and the other concepts needed to do your lessons.
The information on this page may seem like a lot of details to remember, but I promise we’ll get you through the actual math lessons like a breeze! This page is simply a tool that takes the place of a “boring” Glossary of Terms.
Math is a building process. To work with fractions, the student needs, at a minimum, strong skills in mathematical fundamentals including adding, subtracting, multiplying and dividing.Without these basic skills, attempting to do higher level work such as fractions will be very frustrating to the student. If the student is weak in these areas, time is better spent reviewing the basics for additional help.
General Homework Help
The Basic Concept of a Fraction
Before you can make “heads” or “tails” out of fractions, it would be helpful if we first agree that the basic idea of a fraction can be ABSTRACT, unless we name the WHOLE to which we are referring. So it is important to keep this in mind while doing your assignments.
Definition of a Fraction
You might recall that in math a number is a point on the number line. Well, there is a special collection of numbers called fractions, which are usually denoted by a/b, where “a” and “b” are whole numbers and “b” is not equal to “0”.
It may be helpful to get your homework off to a great start by defining what fractions are, that is to say, specifying which of the points on the number line are fractions.
So, here goes…
There are three distinct meanings of fractions —part-whole, quotient, and ratio, which are found in most elementary math programs. To reduce confusion while using this homework helper, our lessons will only cover the part-whole relationship.
The Part-Whole – The part-whole explanation of a fraction is where a number like 1/5 indicates that a whole has been separated into five equal parts and one of those parts are being considered.
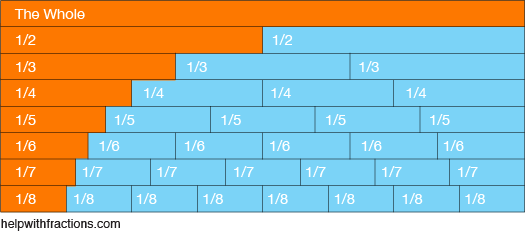
This table is a great help to get a feel of how a fractional part compares to the whole…
As a homework helper, this table shows you how the “same” whole can be divided into a different number of equal parts.
The Division Symbol (“/” or “__”) used in a fraction tells you that everything above the division symbol is the numerator and must be treated as if it were one number, and everything below the division symbol is the denominator and also must be treated as if it were one number.
Basically, the numerator tells you how many part we are talking about, and the denominator tells you how many parts the whole is divided into. So a fraction like 6/7 tells you that we are looking at six (6) parts of a whole that is divided into seven (7) equal parts.
Although we do not cover fractions as a quotient or as a ratio, here is a brief explanation of them.
A Quotient – The fraction 2/3 may be considered as a quotient, 2 ÷ 3. This explanation also arises from a dividing up situation.
For example…
Suppose you want to give some cookies to three people. Well, you could give each person one cookie, then another, and so on until you had given the same amount to each. So,…
If you have six cookies, then you could represent this process with simple math by dividing 6 by 3, and each person would get two cookies.
But what if you only have two cookies?
One way to solve the problem is to break-up each cookie into three equal parts and give each person 1/3 of each cookie so that in the end, each person gets 1/3 + 1/3 or 2/3 cookies. So 2 divided by 3 = 2/3.Here’s a brief explanation of…
A Ratio – A comparison of things as a ratio can be expressed in one of two ways: first, the “old fashioned” method, a:b, pronounced “a is to b“; and second, as found in newer books, a/b. If the ratio of “a to b” is 1 to 4“, or 1/4, then “a” is one-quarter of “b”. Alternately, “b” is four times as great as “a”.
For example:
The width of a rectangle is 7ft and its length is 19ft. The ratio of its width to its length is 7ft to 19 ft, or…
7ft/19ft = 7/19
Since we are comparing feet to feet, we don’t need to write the units.
The ratio of its length to its width is…19 to 7
That was already a lot of homework help and you haven’t worked a problem yet. So let’s put some this stuff to WORK! But remember this is NOT the actual lesson, just a quick overview of some to the RULES and PRINCIPLES we’ll need to use when working with fractions. Don’t worry about memorizing everything, you’ll see all of this “stuff” again as they apply to a particular operation during the homework lessons. So…
We’ll finish up with the…
Rules for Fraction Operations
Adding Fractions
To add fractions, the denominators must be equal. Complete the following steps to add two fractions.
- Build each fraction (if needed) so that both denominators are equal.
- Add the numerators of the fractions.
- The new denominator will be the denominator of the built-up fractions.
- Reduce or simplify your answer, if needed.
- Factor the numerator.
- Factor the denominator.
- Cancel-out fraction mixes that have a value of 1.
- Re-write your answer as a simplified or reduced fraction.
Subtracting Fractions
To subtract fractions, the denominators must be equal. You basically following the same steps as in addition.
- Build each fraction (if required) so that both denominators are equal.
- Combine the numerators according to the operation of subtraction.
- The new denominator will be the denominator of the built-up fractions.
- Reduce or simplify your answer, if needed.
- Factor the numerator.
- Factor the denominator.
- Cancel-out fraction mixes that have a value of 1.
- Re-write your answer as a simplified or reduced fraction.
Multiplying Fractions
To multiply two simple fractions, complete the following steps.
- Multiply the numerators.
- Multiply the denominators.
- Reduce or simplify your answer, if needed.
- Factor the numerator.
- Factor the denominator.
- Cancel-out fraction mixes that have a value of 1.
- Re-write your answer as a simplified or reduced fraction.
To multiply a whole number and a fraction, complete the following steps.
- Convert the whole number to a fraction.
- Multiply the numerators.
- Multiply the denominators.
- Reduce or simplify your answer, if needed.
- Factor the numerator.
- Factor the denominator.
- Cancel-out fraction mixes that have a value of 1.
- Re-write your answer as a simplified or reduced fraction.
Dividing Fractions
To divide one fraction by a second fraction, convert the problem to multiplication and multiply the two fractions.
- Change the “÷” sign to “x” and invert the fraction to the right of the sign.
- Multiply the numerators.
- Multiply the denominators.
- Reduce or simplify your answer, if needed.
- Factor the numerator.
- Factor the denominator.
- Cancel-out fraction mixes that have a value of 1.
- Re-write your answer as a simplified or reduced fraction.
To divide a fraction by a whole number, convert the division process to a multiplication process, by using the following steps.
- Convert the whole number to a fraction.
- Change the “÷” sign to ” x” and invert the fraction to the right of the sign.
- Multiply the numerators.
- Multiply the denominators.
- Reduce or simplify your answer, if needed.
- Factor the numerator.
- Factor the denominator.
- Cancel-out fraction mixes that have a value of 1.
- Re-write your answer as a simplified or reduced fraction.